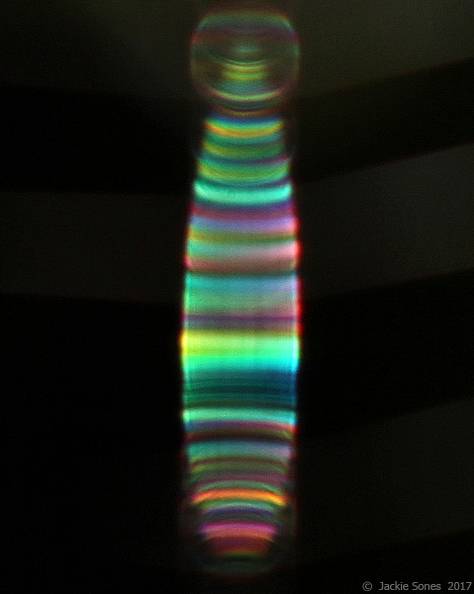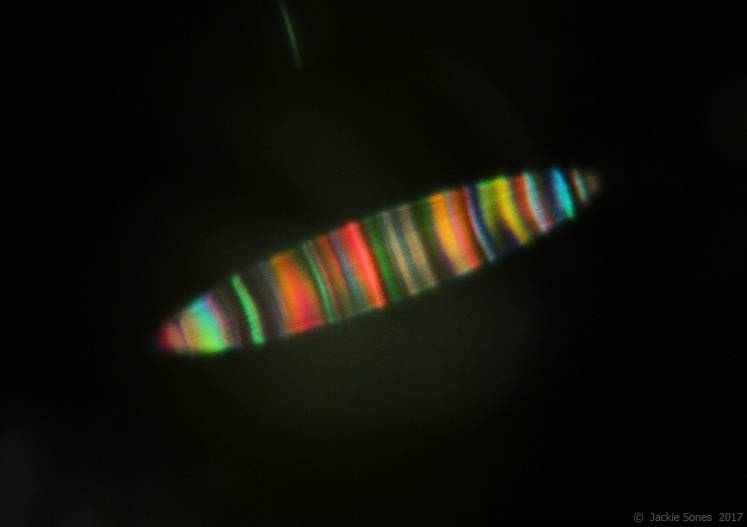

Optical effects on spider webs have already been treated here [1], [2]. There, the main focus was on the colours seen on the sticky threads of orb webs lit from behind. The non-sticky strands show less conspicuous colours to the eye, but recently I saw a series of surprisingly candy-striped out-of-focus highlights in the blog “The Natural History of Bodega Head” by Jackie Sones [3a, b, c, d, e, f] , which puzzled me. Here are two examples (shown with permission):
Why are many of the glints cigar-shaped? Shouldn't one expect that the blurred image of the gloss is everywhere as wide as that of the strand so that the shape would be more or less rectangular?
Fortunately a cellar spider provided the possibility to investigate this. Cigar shaped striped highlights could be obtained, but the results seemingly depend on the camera. The above pictures have been taken with a SLR camera with a 22.5 × 15 mm sensor; my camera's sensor measures 6 × 4.5 mm.
With bare eyes this could not be seen because one involuntarily focuses on the spot where one looks, and then most of the colours vanish. But even if one succeeds to focus on the far background with a glistening silk strand nearby, the colours will not be as beautiful as in a photograph. With the camera arbitrary defocussing is easy, and moreover, details of a high resolution image can be enlarged. The larger the sensor of a digital camera, and the larger the aperture, the more impressive are the results.
When focused to infinity, so to say to the sun's one-dimensional mirror image, the highlights are only thin stripes orthogonal to the threads. The width of the coloured stripes is the apparent diameter of the sun, their length is the apparent breadth of the out-of-focus strands (which are not seen in the right picture except for their glints). The closer the strands, the longer the stripes. If, however, the strand is focussed at, the (one-dimensional) image of the sun gets blurred and the gloss is seen on a longer stretch which is given by the apparent diameter of the blurred sun.
The colours are due to the fact that the surfaces of the silk strands are not smooth, but slightly wrinkled. If out of focus, light arriving at one point of the sensor comes from nearby points of the strand and has travelled different path lengths because of the wrinkles. Interference can result in extinction of parts of the spectrum and enhancement of other parts. This is perceived as colour.
But what is the reason for the peculiar shapes of the highlights?
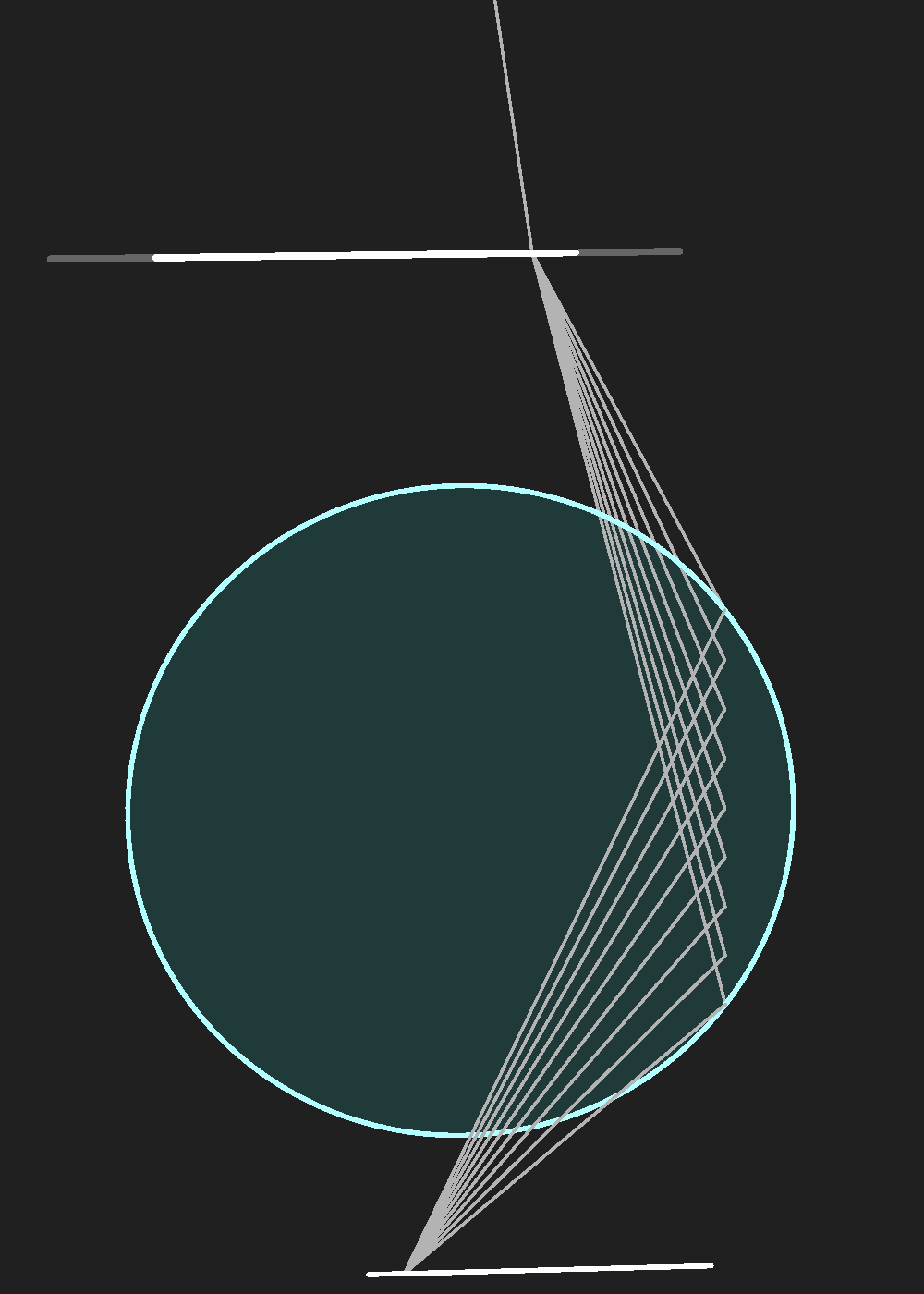
We assume the simplest possible geometry. The silk strand is assumed to be horizontal and the incoming rays of the sun are perpendicular to it. A side view and a view from above are shown below. In the side view, the silk strand is seen as a point. By reflection, the light rays are fanned out and meet an ideal, very thin lens at distance a. The rays are refracted by the lens and meet at the image of the strand.
In the horizontal projection we see that the rays reflected by the thin glossy line all include the same angle with the line as the incoming rays, which is 90° in this case. Therefore, all the rays pass a vertical line through the focus of the lens.
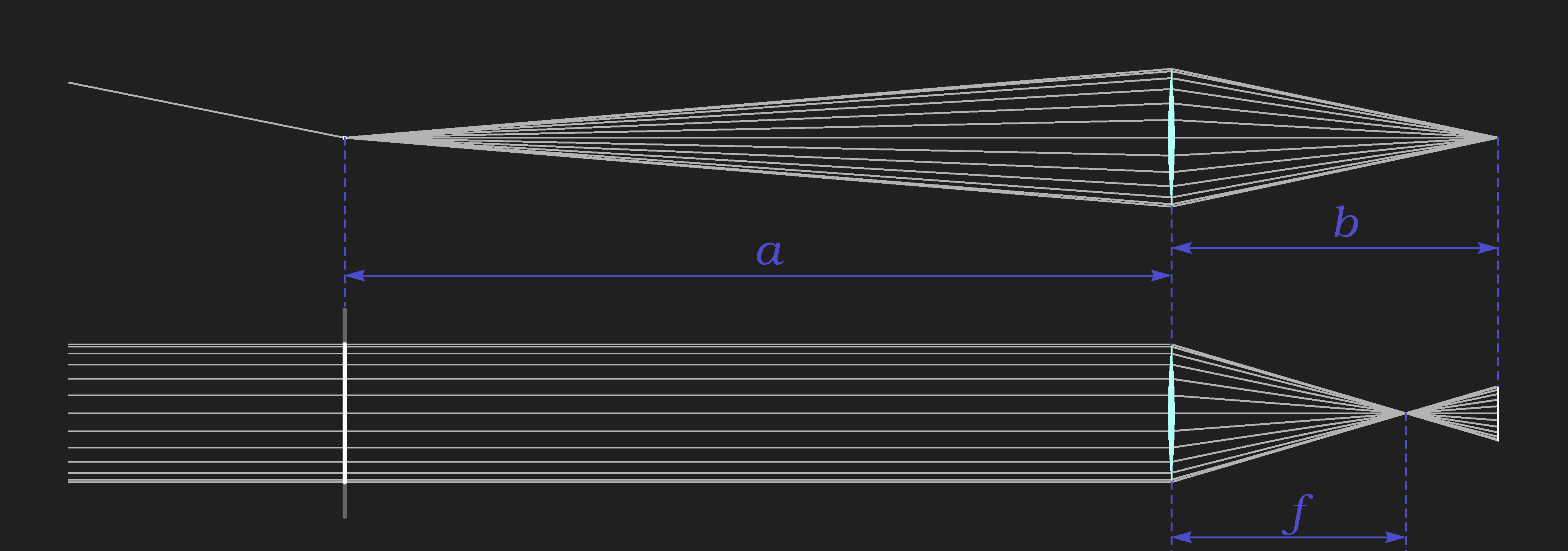
Here and in the following we speak of “reflection” of the light. Perhaps the terms “deflection” or “scattering” would be more appropriate, as refraction and diffraction contribute as well. However, for the geometrical considerations this is not important. Notwithstanding that diffraction and interference effects are clearly visible, we ignore them for the moment and use geometrical optics to explain the shape of the glistening out-of-focus specks.
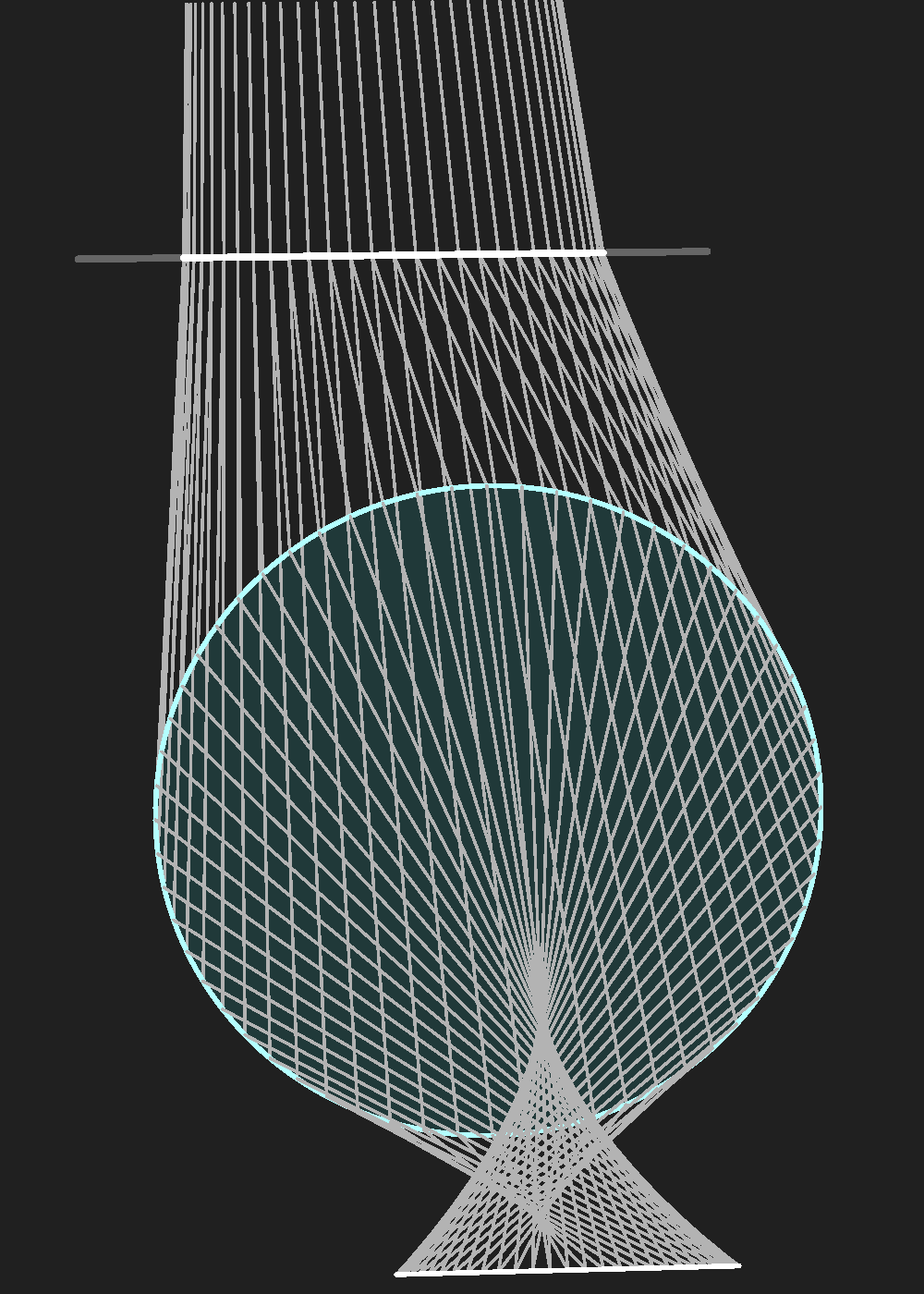
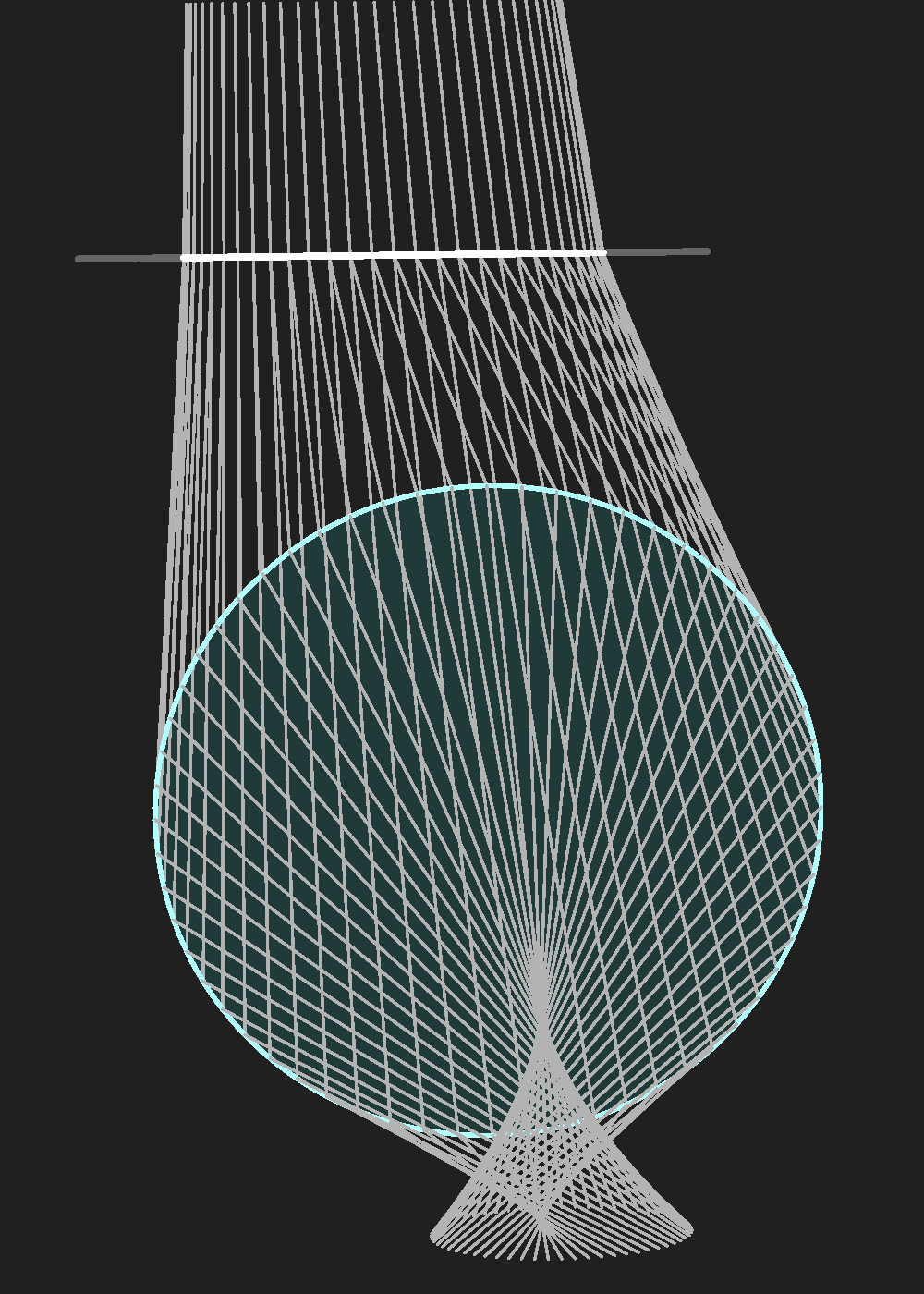
To the right, there are perspectivic views of the same arrangement, as seen from the side of the photographer. In the first sketch, only one ray from the sun is considered which is reflected by the glossy line. The reflection condition is, that the reflected rays all include the same angle with the strand as the incoming ray, which in this case is 90°. Therefore, all the rays from one point of the strand hit the lens along a vertical line, where they are refracted to meet in one point of the strand's image. In the second sketch, only those of the reflected rays are drawn which pass the lens just at the rim (of the opening of the diaphragm which is not shown). The rays are drawn up to the point where they meet to form the image of the thread, a horizontal line. There the camera's sensor would be, if it were focused on the thread. If, however, the sensor would be slightly closer to the lens, the outline of the gloss would be a slender ellipse. To show this, in the rightmost picture the rays are stopped somewhat before they would meet.
Reducing the distance between lens and sensor more and more, the width of the ellipse would decrease while the height would increase until, when the distance is the focal length f, i.e. when focusing to infinity, it becomes a vertical line.
If the sun were much smaller, “pointlike”, then the highlights in the fourth photograph above would be thin lines. For simplicity, this has been assumed for the schematic sketches here.
If we now consider the sun's disc, we have, loosely speaking, to superpose many of such elliptic discs which are at different positions along the image of the thread. But the ends are still rounded, not pointy. Acute tips can result when the diaphragm is not fully opened, but forms e.g. an hexagonal opening. Then the cross section is a squeezed hexagon.
If there is sufficient ambient light in sddition to direct sunlight, then the rest of the thread is imaged as a dim blurred stripe of constant width.
Diffraction effects are the more pronounced the smaller the aperture is. The first photo in the second row above has been taken with 8.4 mm focal length and aperture f/4, that is 2.1 mm, and the width of this cropped image on the sensor was 1.8 mm. Dark lines parallel to the strand in the highlights are due to Fresnel diffraction, as well as the diffuse outlines of the highlights.
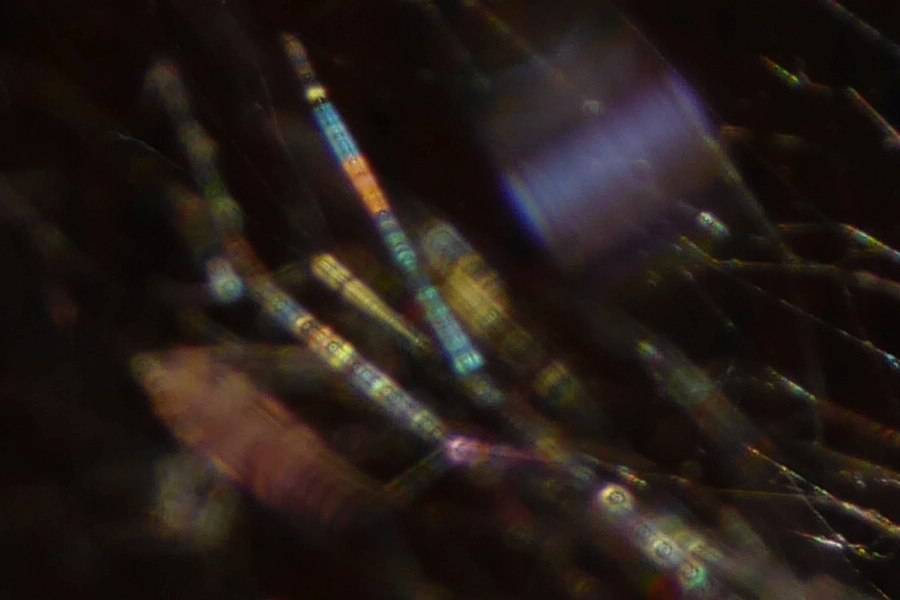
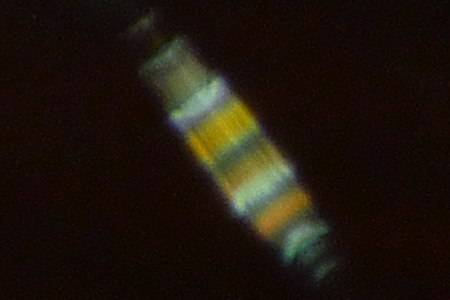
The threads of the webs of the Pholcidae (cellar spiders) are not sticky, thus there are no regular arrays of glue droplets which might act like a diffraction grating. But on a microscopic scale the strands are slightly wrinkled or puckered, or at least their diameter staggers slightly. Therefore, the waves outgoing from the glossy strand are not purely cylindric. The puckers may be irregular or to some extent exhibit some regularity like a nearly periodic repetition. The wavelets (in the sense of the Huygens-Fresnel principle) arriving at one point of the sensor from nearby parts of the strand may interfere constructively or destructively as the distances which they travelled vary. Nice examples of quite chaotic sequences of colours have been given in the beginning; to the right there is an example with surprising regularity in the lower part (from the same source [3c]).
The larger the aperture, the longer are the images of the strands' highlights, and correspondigly there appear the more coloured stripes, if the thread is not too much out of focus. Focusing to larger and larger distances, more and more stripes merge until, when focused to infinity, each highlight is imaged as a single stripe.
In the geometrical optics picture, and in the simple geometry sketched, if the thread is out of focus, every point of the thread is imaged as a line orthogonal to the thread. Accounting for interference, we would therefore expect coloured stripes orthogonal to the direction of the strand. This is, in fact, approximately the case, but not exactly. In the middle of the highlights fairly well, but near both ends, the coloured bands are bent. If the thread is blurred because it was too close to the camera, the highlight is striped like )))|||||(((, but if it was too far, the pattern is like (((|||||))). Examples are shown below:
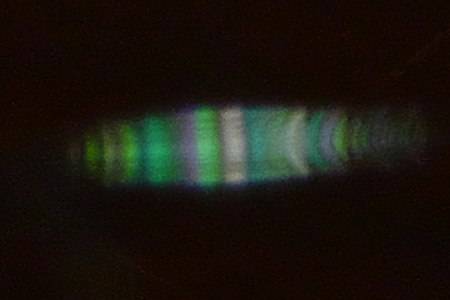
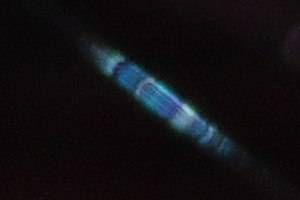
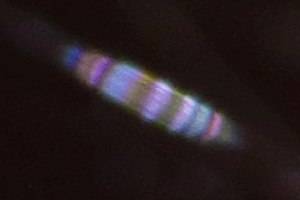
An ideal simple lens, which maps every point of the object plane at arbitrary distance to a point in the corresponding image plane, does not exist. Photographic objectives are designed to achieve this goal without distortion of the image to a good approximation, but the ray paths behind the lenses are not as simple as implied by the above sketches.
If the silk strands are very irregular, of varying thickness etc., then there may be Fresnel diffraction patterns of pointlike glints superposed over the striped image. These may be due to very small portions thicker and glistening much brighter than the neighbouring parts.
Moreover, except direct sunlight, there is diffuse ambient light, and so the nonglossy parts of the strands are visible too in the images. All this can be seen in the next pictures. The last one (from [3f]) shows part of an orb web. Apparently at the points where the catching threads are fixed to the radial strand there are larger droplets of glue, their glints causing circular Fresnel patterns.