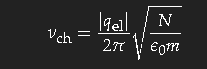Crystals, minerals,
metals, pigments
Azurite, a basic copper carbonate mineral, had been used since ancient times to produce blue pigment, and has only recently been replaced by synthetic blue pigments.
Traces of sodium brought into a flame emit bright yellow light colouring the flame. Light of the same wavelengths as can be emitted can also be absorbed by sodium atoms. Nevertheless, sodium vapour is not coloured; only by spectroscopic means the absorption can be observed at all.
Metallic sodium is never found in nature. The weakly bound valence electron is easily “stripped” off. In a solution of table salt sodium is ionized, the electrons of the Na+ ions “sit” in very stable closed shells (rare gas configuration), and the energy of photons of visible light is too small to excite the ion. The electrons given off by sodium are attached to the chlorine ions, completing the stable noble gas configuration of Cl–, which is colourless for the same reasons. Therefore, table salt solution is as clear as pure water.
To excite the water molecules, ultraviolet radiation is necessary – clean water is colourless. Only in thick layers one can see the weak blue-green colour due to little absorption going along with excitation of molecular vibrations.
Quite generally one realizes that compounds of elements from the first three rows of the periodic table are colourless as long as the molecules are small, consisting of only few atoms.
Colouring ions
With increasing nuclear charge and accordingly increasing number of electrons, the atomic level scheme gets more and more involved. In the following we consider the properties of an only partially filled 3d shell, as it occurs in the transition elements chromium, manganese, iron, cobalt, nickel, and in copper (II) ions.
In a crystal field
Among the heavy elements, in particular the ions of the transition metals absorb visible light if they are part of a crystal lattice.
While in the ideal case of an isolated ion the electrons feel only the forces due to the nucleus and the other electrons of the same ion, in a crystal the presence of neighbours causes additional fields of force. The interaction with the neighbours causes shifts of the energy levels which depend on the spatial orientation relative to the surroundings. In addition, instead of sharp levels narrow energy bands are formed, as will be described later.
Qualitatively the effect of a crystal field may be visualized in our water-cup model. If the cup were squeezed so that its cross-section became oval, swashing along the short axis will have a higher frequency than that along the long axis.
 |  | | Other oscillation modes are affected too, depending on their shape.
Double-clicking the images will swith on the animation, a single click switches it off again.
To start the animation in a separate window, click on the symbol ◊ under the respective picture.
|
◊ |
◊ | |
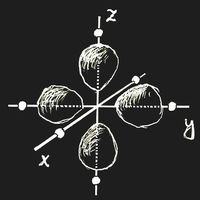
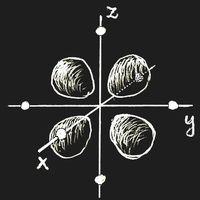
Think of an ion with a single 3d electron, Sc2+ or Ti3+, which is placed in a crystal lattice. (We choose the origin of the coordinate system at the site of this ion.) In an important and frequent case the ion is surrounded by six negative ions, all in the same distance located on the coordinate axes. This is called an octahedric configuration: the surrounding anions are at the corners of a regular octahedron centred at the origin.
 | |
 |
| (a) | | (b) |
If there is an external field, the energy of an ion depends on the orientation of the electron clouds. Let the ion have six anions in an octahedric configuration as neighbours. If the partial clouds of the 3d orbit point towards the nearest neighbours, as in figure (a) above, the energy will be higher than in the case of figure (b), where the electron density is zero along the coordinate axes. This energy difference is called Δ. Of course, the field due to the neighbouring ions will also change the wave function itself, but in first approximation this change may be neglected.
In the ground-state, the single 3d electron will occupy a state of type (b), and there is the possibility that light of frequency ν = Δ/h induces a transition into one of the higher states while radiation energy is absorbed.
Next we consider an ion with more than one d electron. The electrons repel each other, and this repulsion leads to an energy splitting of states with different total orbital angular momentum (“gross structure”). In addition to that, there is an interaction between spin and orbital angular momenta which induces further splitting depending on total angular momentum (“fine structure”). For the light elements, spin-orbit interaction is small.
As long as there is no external field there is no preferred direction in space, angular momentum is conserved and quantum states may be labelled by angular momentum quantum numbers.
Empirical rules summarize the influence of the Coulomb interaction of electrons in one shell:
Hund's rules:
– Full subshells and shells have total angular momentum and total spin zero.
– In the lowest energy state, the total spin S takes on its maximum value (thus the electron spins tend to be parallel)
– Among the states with the same spin, the one with largest orbital angular momentum has the lowest energy.
– A shell which is less than half filled has J = | L – S |;
if it is half filled or more, then J = L + S in the lowest state.
The ion Cr3+ has three electrons in the 3d shell (in addition to the closed shells). To find the ground state according to Hund's rules, one looks for the state with maximum z-component of total spin and orbital angular momentum.
The three single spins are parallel, thus S = 3/2. According to the Pauli principle, the single-particle states with m = 2, m = 1 and m = 0 can be occupied by one electron each, the sum is M = 3 and therefore L = 3. In spectroscopic notation the state so obtained is denoted by 4F. The left upper index gives the multiplicity of spin orientations 2S + 1, the letters S, P, D, F, . . . (capitals for composite systems) stand for L = 0, 1, 2, 3, . . .
For the next higher level Hund's rules give the multiplet 4P.
Corundum, Al2O3, and beryl, Be3Al2Si6O18 are naturally clear. If aluminium ions are replaced by chromium (III) ions (few percent), corundum becomes red and is then called ruby, while beryl becomes green emerald. In both cases the chromium ion is surrounded by six oxygen ions in slightly distorted octahedric configuration, but because of the different chemical composition the crystal field is slightly weaker in emerald than in ruby. What happens to the F multiplet in an octahedric crystal field?

 Left:
Left: Ruby,
right: Emerald. Both in ruby and in emerald the colour is due to chromium ions which substitute few percent of the aluminium ions. Both pictures (taken at a Gem and Minerals fair) magnified ca. 2.5 times.
The symmetry properties of three electrons in an F-state are the same as those of a single f-electron. Among the single-particle f-functions there is one which is transformed into itself by all symmetry operations which leave an octahedron unchanged (changes of sign being irrelevant). It may be written as xyzf(r) and the corresponding density distribution consists of eight small clouds, the centres at the corners of a cube. By this, the ions sitting at the corners of an octahedron are optimally avoided, and therefore the energy of this state is lowest. However, that the remaining six f-wavefunctions combine to form two triplets, cannot easily be seen.
 | | 
|
| Ruby | | Emerald |
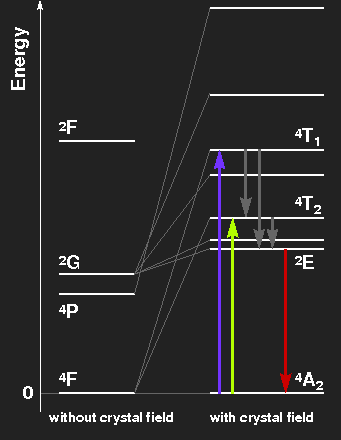
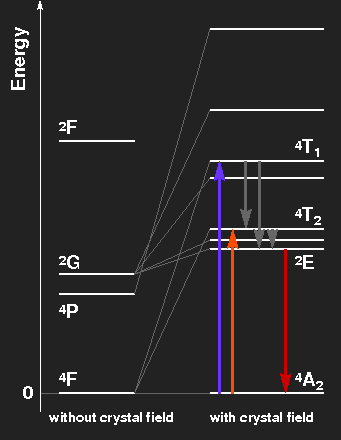
Level scheme of the Cr3+ ion, showing the splitting of levels by the crystal field. The level spacings correspond to ruby on the left side, to emerald on the right.
Ruby: Violet and green light are extinguished, thus the transmitted light consists mainly of red light with a little blue. The electrons in excited levels give off their energy by emission of infrared radiation and by thermal interaction with the crystal lattice. By these mechanisms, the level labelled 2E is reached. In transitions from there to the ground-state, red fluorescent light is emitted. This has nothing to do with the colour of the gemstone, but the light of ruby lasers comes from this transition line. Transitions from the ground-state to the levels originating from the 2G state are highly improbable because a spin-flip would be necessary.

 Fluorescence of ruby:
Fluorescence of ruby: Left: Ruby in parent rock (from Froland, Norway), in daylight.
Right: Same specimen irradiated by ultraviolet light (“black light”).
Emerald: The slightly weaker crystal field splits the levels a little bit less. The absorption maxima are in the orange and blue-violet region, green light is transmitted. The red fluorescence line is much the same as in ruby.
The spreading of the levels to form energy bands (see below) has been disregarded.
Admixtures of iron and titanium cause corundum to become blue sapphire.
Chrysoprase (a variety of chalcedony) gets its green colour from the presence of small quantities of nickel, whereas the brown or red colour of quartz pebbles is due to iron ions.

| Amethyst is a purple variety of quartz. Its colour is due to foreign atoms (iron according to Wikipedia; However, the exact structure of the colour centres seems to be not yet resolved). The figure shows part of an amethyst geode from Brazil, ca. natural size. |
The colours of crystals which are due to traces of foreign atoms are only conspicuous because the crystals otherwise would be clear. They are, so to say, only weakly coloured, if they were pulverized, no colour would be seen. The powder colour (also called streak) is used as a diagnostic tool for mineral identification, and it is white for this class of minerals.
Pleochroism
Pleochroism, “multicolour” (dichroism, trichroism) can be observed on some anisotropic crystals. The best known example probably is the tourmaline.
The three pictures show a small fragment of a tourmaline crystal (L=28 mm), taken without filter (top), and with a polarizing filter, polarization (electric field) parallel to the crystal axis (middle) and perpendicular to the axis (bottom).
Tourmalines often show different colours in one crystal, the specimen shown is blue in the left third and green otherwise. This, however, is not meant by the term “pleochroism”, but the phenomenon that the colours are different for different polarizations of the light, as shown by the lower two images.
As has been discussed in the cases of ruby and emerald, embedding the colouring ion in a crystal field leads to a splitting of its energy levels. The lower the symmetry of the crystal field, the more complicated is this splitting. In the case of green tourmaline, green light is not absorbed if the electric field is parallel to the symmetry axis (“extraordinary ray”), but strongly absorbed if the field is perpendicular (“ordinary ray”).
Tourmaline (trigonal crystals) is optically uniaxial and therefore dichroic, other crystals with more than one optical axis may show trichroism.
In former times, small plates cut from tourmaline have been used to investigate the polarization of light; today polarizing filters are made from plastic foils doped with iodine and stretched, thus artificially inducing strong dichroism.
Complexes
The more involved structure of the heavier elements, particularly the transition metals, allows more involved kinds of chemical bonding.
The lowering of some energy level through the interaction with neighbouring atoms, molecules or ions means that for the system as a whole the energy is lowered by the interaction which keeps the constituents together. As all parts obey quantum mechanics, the wave functions arrange themselves in such a way that in the ground-state the energy is a minimum. In good approximation, the molecular wave functions (orbitals) can be viewed as superpositions (linear combinations) of the orbitals of the participating atoms or ions, and binding as well as antibinding linear combinations can be formed in the same way as in the well known case of covalent bonds.
If a shell below the valence shell is involved in the bonding, the results are called complexes. The atoms, ions, or molecules bound in that way to the metal are called ligands. Typical ligands are halogenide ions, OH –, H2O, NH3, NO2–, CN –.
Essential for the colour of metal complexes is the splitting of energy levels (which in the spherical case all have the same energy) by the electric field of the ligands, making transitions within the same subshell possible, and on the other hand the change from atomic orbitals to much more extended molecular orbitals with the consequence of much larger absorption probabilities. (The connection between size and absorption and emission probabilities has been discussed in the section on dyes.) Distortion of the wave functions and small admixtures of waves with different symmetry can lead to transitions which otherwise were forbidden by symmetry (“selection rules”).
 | In copper(II) sulfate CuSO4·5H2O the five water molecules are differently bound. Four of them are coordinated to Cu2+ in a planar square configuration; they are lost when the salt is heated to 100ºC; the fifth one is bound to the sulfate anion by hydrogen bonds and is given off at 150ºC or higher.
When the crystals are dehydrated they turn from blue to greyish-white.
|
| Copper(II) sulfate (crystal fragments) |
Only the basic ideas could be sketched here; for more thorough discussions and explanations of chemical bonds and ligand field theory, textbooks of chemistry and solid state physics should be consulted.
We should mention one mechanism which in many cases is active also in complexes:
Charge transfer for strong absorption
Compounds which contain the same element in different oxidation states are frequently coloured, which means they can strongly absorb light. As an example we take magnetite, FeO·Fe2O3. According to the chemical formula one unit of Fe3O4 consists of one Fe2+ ion, two Fe3+ ions and four O2– ions. But in quantum mechanics states are possible where one electron is distributed over three Fe3+ ions so that one cannot tell which of them is two-valued. There is an analogy between wave-functions and the vibrations of a chord. There is the lowest-frequency vibration corresponding to the ground-state, and there are higher harmonics where some parts of the chord move in one direction and other parts in the opposite direction. In a superposition of ground- and first excited state, the charge oscillates strongly over a comparatively large distance. This indicates that under the influence of an electromagnetic wave, transitions between these two states are likely to occur, which amounts to strong absorption. This property persists if many units join to form a crystal; in a crystal lattice the spreading of energy levels to form energy bands greatly extends the range of absorption.
 |
Magnetite crystal in matrix. Magnetite can absorb light of all frequencies and therefore is black, its streak is also black, it is strongly “coloured”, though not in a vivid hue. Synthetic magnetite powder is traded as a pigment (iron oxide black). |
Charge transfer does not only happen between ions of the same element: the blue colour of sapphire is due to charge exchange between iron and titanium ions. Sapphire is a variety of corundum. The two kinds of impurity may be present as Fe2+ and Ti4+ or, with higher energy as Fe3+ and Ti3+ in the crystal lattice. The state of lowest energy of an iron – titanium pair is a superposition of both, such that the iron has somewhat more than two positive charges, the titanium accordingly somewhat less than four, one electron being shared by both in a “bonding state” where the sign of the wave function is the same at both sites (Fe and Ti). A similar “antibonding” state where the sign is different has somewhat higher energy and can be excited while a photon is absorbed.
Transitions betweel bonding and antibonding molecular orbitals may be viewed as charge transfer, this can cause strong colour of complexes if the transition frequencies are in the visible region. However, discussion of level schemes of complexes is beyond the scope of this article.
 |

|
| Malachite, Cu2CO3(OH)2 | Azurite, Cu3(CO3)2(OH)2 |
Malachite and azurite pigments had been used since antiquity. Today they are needed only for restoration of old paintings.
Solids
What happens when atoms join to form molecules or solids is mainly determined by the fact that particles also exhibit the properties of waves. The peculiarities of waves and oscillations, on the other hand, are quite general and depend little or not at all on the substance which oscillates or sustains the waves. Therefore, many features of microscopic systems can be seen in macroscopic oscillations or waves.
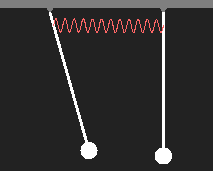
Every possible state of an electron in a simple atom corresponds to a wavefunction, as we have discussed. Stationary states oscillate with fixed frequency. We consider a pendulum as an analogue of an atom in a certain quantum state. Another atom in the same intrinsic state be represented by a second pendulum of the same oscillation frequency. If two atoms come close to each other, they will interact due to the electric charges contained in them. We simulate this interaction by a weak spring. What is going to happen?
 | | Animated pictures on these pages may be switched on by double clicking; a single click switches them off again.
To start the animation in a separate window, click on the symbol ◊ under the respective picture.
Motion of two coupled pendulums after initial displacement of only one of them. |
◊ | |
We are particularly interested in states with harmonic motion (which means that all parts of the system perform sinusoidal oscillations with the same frequency and phase), because they correspond to quantum states with constant energy according to E = hν. Without coupling, both pendiulums swing with equal periods. With coupling, there are only two simply periodic types of motion with slightly different frequency. In the first case, the pendulums swing in phase with the same speed as a single one, as the coupling spring is neither expanded nor contracted. In the other case the pendulums move opposite to each other, the coupling spring increases the restoring force and the period is shorter.
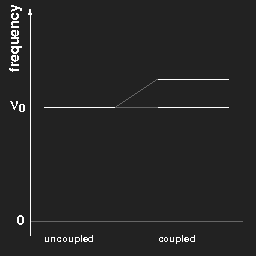
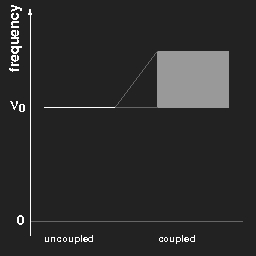
Plotting the frequencies in a diagram, we obtain for two pendulums a kind of “level scheme” as shown in the left figure.
 Left:
Left: The frequencies of two equal pendulums without and with coupling.
Right: the same for a chain of “infinitely” many pendulums.
For (a chain of) coupled pendulums the lowest frequency is the same as that of a free pendulum. This is a special case and does not hold for arbitrary systems.
In the case of three pendulums there are three different harmonic modes and three different eigenfrequencies; for a chain of N coupled pendulums one gets N different frequencies, and when N becomes very large, “tends to infinity”, the limiting case is a continuous band of possible frequencies ranging from νmin to νmax, as shown in the right figure above.
Qualitatively, the quantum states of atoms show the same behaviour when atoms come close to each other. We have to bear in mind that the energy is proportional to the frequency, and that the same considerations apply to each energy level.
Think of a crystal built by adding one atom after the other, and consider the possible energies of the most loosely bound electrons (neglecting the mutual interaction for the moment). For one isolated atom we have a level scheme with discrete energies, and in the ground-state, the lowest levels are occupied by electrons. For each of these single-particle levels holds what we have seen in the case of pendulums, namely a splitting of the possible energies into more and more neighbouring levels which in the case of “infinitely” many atoms join to form energy bands.
Such a band structure results also in the case that two or more kinds of atoms make up the crystal. As the more closely bound electrons are less affected by the influence of the neighbouring atoms or ions, the spreading of the lowest lying levels is small; for the strongly bound electrons of the ion core there are only small changes.
After all, the Pauli principle holds.
| Now look at a crystal of table salt (sodium chloride, NaCl). The sodium ion has the same electron configuration as the noble gas neon, the chlorine ion the same as the noble gas argon. These configurations are very stable, to induce excitations, more energy is needed than the photons of visible light can supply. Though the energy levels of sodium and chlorine are spread to form bands, there is still a large gap between the highest occupied and the lowest empty levels. Light cannot be absorbed, a pure crystal of table salt is clear and colourless.
| |

Rocksalt crystals from Wieliczka, Poland (Museum of Natural History, Vienna) |
Crystals formed from atoms with only partially filled outermost shell are quite different. Empty energy levels are very close to occupied ones. Little energy is needed to change the electron distribution. The electrons can move almost freely within the crystal – typical metallic properties. The optical properties of metals shall be discussed later.
Electric conductivity is the distinctive property of metals. Electric current in a solid is only possible if not all states of an energy band are occupied. This is plausible: The moving electrons carry electric charge, but if all levels are filled, the amount of charge carried from left to right is exactly the same as that moving in the opposite direction. This is not changed if an electric field is applied, since, due to the Pauli principle, no redistribution of electrons is possible. If, however, a band is only partially filled, the electrons are free to change their direction of motion and a net current can flow.
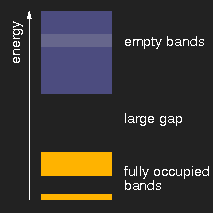 |
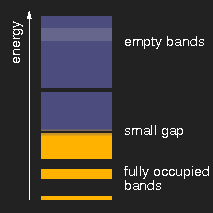
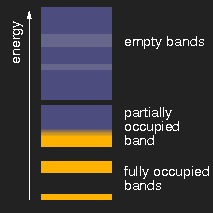 |
|
| insulator | metal | semiconductor |
Schematic drawing of typical band structures. (The lighter areas in the empty bands mean that there is an overlap of bands originating from different levels.)
Depending on the size of the energy gap between the last filled and the first empty band or, respectively, the presence of partially filled bands, the solids are insulators, metals, or semiconductors.
Band structure and colour
The optical properties are also determined by the band structure. In insulators like diamond, table salt, ice, sugar, calcite and many others, there is a large gap between the last occupied and the first empty levels. Photons of visible light do not have enough energy to lift an electron across this gap, and therefore cannot be absorbed, and the crystals are colourless and clear, if they do not contain impurities.
Other substances, still insulators, have a somewhat smaller band gap, so that the shortest-wavelength (highest energy) visible photons can lift electrons from the lower to the upper band and thus can be absorbed. These substances are yellow. Examples are sulfur, cadmium sulfide (CdS, the mineral greenockite, the pigment cadmium yellow), orpiment As2S3.
 |  |
|
Sulfur | Cadmium yellow light, cadmium yellow medium, Cadmium orange (watercolours) |
It is interesting that cadmium sulfide and cadmium selenide can form mixed crystals in any proportions. The band gap depends on the amount of selenium present, and with increasing selenium percentage, the colour changes from yellow over orange to red, brown and finally
black.
In contrast to the substances which are colourless when pure and coloured due to foreign atoms, the crystals dealt with here are “strongly” coloured, the powder or streak is coloured too. This qualifies such substances for the use as pigments.
If finally the band gap is so small that it can be overcome by thermal energy, the substance becomes a semiconductor. Graphite is an example. If the gap vanishes at all when the bands overlap, the substance is a metal and has the well known metallic appearance.
Lustre and colour of metals
The electrical as well as the optical properties of metals are due to the existence of conduction electrons, which means the electrons in a partially filled energy band which can move almost freely.
From the band structure we have seen that a metal can absorb electromagnetic radiation from the infrared to the ultraviolet. One might think that metals should therefore be black.
| |
 |
Fine metal powder is indeed black, but as a consequence of electric conductivity, larger pieces of metal reflect light, metals are shiny and the brighter the better they reflect light.
For the following, details of the band structure are not important, and we adopt the simplest model for a metal, namely a free electron gas, the charge of which is compensated by the lattice of fixed positive ions.
Due to the good electrical conductivity, any potential differences between different points will be compensated rapidly by electric currents. Inside of an ideal conductor (with infinite conductivity i.e. no resistance) no electric fields can exist.
What happens if an electromagnetic wave hits an ideal conductor? At the surface of the conductor, the electric field must be zero. We can use a mechanical model to see what happens.
If an end of an elastic rope is fixed and a wave is travelling towards this point which cannot move, the wave will be reflected, changing its sign as shown in the animated picture below:
 ◊
◊
In a calculation, the fulfilment of the boundary condition can be achieved by adding a wave which propagates in the other direction and is the negative of the incoming wave.
The better the conductivity, the higher the reflection. The lustre of metals is a consequence of their electrical conductivity!
Silver and aluminium, both very good conductors, reflect much more than iron. For the yellow light of the sodium spectral line (589,3 nm), the reflection coefficient of the three metals mentioned is
94%, 83% and 33% (after Born & Wolf 1975).
Of course, under normal conditions, the conductivity is never infinite. In particular, the inertia of the electrons due to their mass inhibits an immediate quenching of the electrical field, and for higher frequencies this inertia becomes more and more important. Above a certain frequency, the properties of the metal become similar to those of an insulator, which means that it becomes transparent and its reflection coefficient decreases accordingly.
With simple model assumptions one obtains the characteristic frequency for this transition
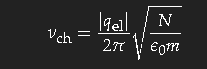
(qel is the charge, N the density and m the mass of the carriers – electrons in a metal, ions in an electrolyte). This formula gives the correct order of magnitude; the exact value depends on subtle details of the band structure of the metal.
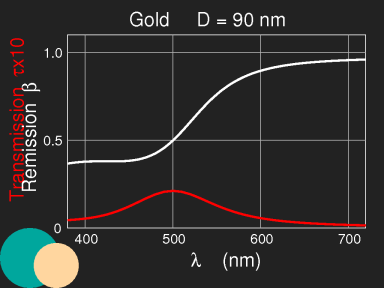
The colours of copper and gold are due to the fact that long-wavelength light is better reflected than the short waves, which penetrate deeper into the volume and get absorbed there. The characteristic frequencies of these metals are in the optical region; thin gold foils look blue-green in transmitted light.
A solution of table salt (sodium chloride) is clear though it conducts electric current. However, the characteristic frequency is so low that its optical properties are like those of an insulator. This holds generally for electrolytes.
Metallic nanoparticles
If metals are finely dispersed in colloidal solutions, they produce colours which differ from those of the bulk metal. Gold ruby glass, also known as cranberry glass, stained by submicroscopic gold particles, probably is the best known example. It contains only 0.01 to 0.02 weight percent of gold.
Gold is added to the molten glass in the form of “gold chloride”, i.e. chloroauric acid, HAuCl4, the product formed when gold is dissolved in aqua regia. After rapid cooling, the glass is still colourless transparent; monovalent gold ions are present in this stage (F.E. Wagner et al.). Heating the glass again to about 600°C, the gold particles aggregate to small clusters.
 |
Left, front: ruby glass in the dip-overlay technique,
middle: dip-overlay caps made from pure ruby glass, clear before and ruby red after re-heating,
back: glass coloured with silver nanoparticles.
Manufacturer: Nachtmann
Photo: Deutsches Museum
|
Colour of gold ruby glass, computed for spherical gold particles with radius 7 nm (experimental data as above:
http://refractiveindex.info/). The scale gives the thickness, multiplied by the density of particles and by the cross section of one particle. For details, see the section on
Mie scattering.
A gaslike mixture of positively and negatively charged particles is called plasma. The electrons in a metal, the charge being compensated by the ionic lattice, is a special case of plasma. In plasma waves or plasma oscillations the positive and negative charges move collectively against each other. The characteristic frequency νch which we encountered above is just the frequency of plasma oscillations in the bulk metal.
The dipole oscillations are easily excited by electromagnetic radiation. Electrical resistance provides the damping – thus the radiation is absorbed and transformed to heat.
The complex index of refraction accounts for these microscopic processes phenomenologically.
The strength of absorption has been dealt with in the section on dyes; there we have seen that the size of the particles is decisive as long as the particles are small compared with the wavelengths of light.
Colour centres, lattice defects
There is one more mechanism by which clear crystals may become coloured, namely point defects in the crystal lattice. Vacancies in ionic crystals are called F-centres, where “F” stems from the abbreviation of the German word “Farbe” (=colour).

| Cleaved fluorite octahedra. Fluorite, CaF2, occurs in different colours which are presumably due to crystal point defects. These can be caused by radioactive irradiation or by impurities. |
Consider the simplest case of a vacancy in an alkali halogenide crystal. We assume that there is a slight surplus of the alkali metal in the crystal, or, in different words, some halogenide ions are missing, leaving their site vacant. As the crystal as a whole is electrically uncharged, there are extra electrons present, one for each vacancy. (One might think that the surplus metal atoms are not ionized. But being part of the lattice, there is no difference between them and the other alkali ions, they are ionized as well.) The additional electron is held in the vicinity of the vacancy by electric forces, there is, so to say, a bound state of a vacancy and an electron.
Now the reasoning is the same as in the case of atomic spectra. The electron is described by a wave function. Corresponding to its different modes there are different discrete possible energies; the lattice defect behaves like some quasi atomic structure within the crystal lattice. The levels of this quasi-atom in the gap between the energy bands of the insulator open new possibilities of transitions involving photon absorption and emission. The excitation energies of the electron bound to the lattice defect are in the range of visible light, therefore they give colour to the otherwise clear substance.
As missing electrons in a crystal lattice behave like particles (“quasi-particles”, “holes”), the situation is very similar in the case of a surplus of halogenide ions. Similar situations arise when in a lattice some ions are replaced by other ones with different charge: some surplus charge at a lattice site and an electron or hole loosely bound to it.
Neighbouring lattice defects may form quasi-molecules, in particular defects of different types. This yields many different possibilities of colour generation.
Luminescence (fluorescence and phosphorescence)
When discussing ruby we already encountered fluorescence. Transitions between energy levels due to absorption of a photon can also occur in the other direction with emission of a photon. However, as in the case of ruby, there are other concurring ways of de-excitation: the energy may be transferred to lattice vibrations or may be radiated off faster by infrared radiation than by visible light. Therefore, under most conditions, light emission by cold matter is not seen.
If energy is supplied to create excited states which can give off their energy by radiating light then luminescence is observable. This is treated in a special section.
Pigments


Pigments in a store for artists' supplies. From left to right: permanent light yellow, yellow ochre, iron oxide red (English red ?), iron oxide yellow, raw sienna, chrome green.
Strongly coloured minerals can be used as pigments if they are not water soluble and sufficiently resistant to weathering. Some of them have been used as examples to illustrate the mechanisms responsible for colour.
You may find in the Web compilations of origin and historical development of anorganic pigments, listings of common pigments which are used for painting and production of paints and inks, and of the chemical composition. A good starting point for the search is Wikipedia.
Back to the index page “the origins of colour”